R2F(1/2B)
Robert P. Munafo, 2023 May 25.
-, ,
.)`
-*_,. -b
_@),c-de.
' . -, .'^@"F*^*
'`,, ., 'b . ^ac.^d^
.____________e______L""`e_____a____\________a_a_ev_d__)aF"^`
` -"-r` ' `% '` ' ' ^^ -` "`@v-[
.` ' '" a~`.-*m-_
' _arFrF$@Y
.:F\ `:$`
_/d` "
.,*-
'
|
|
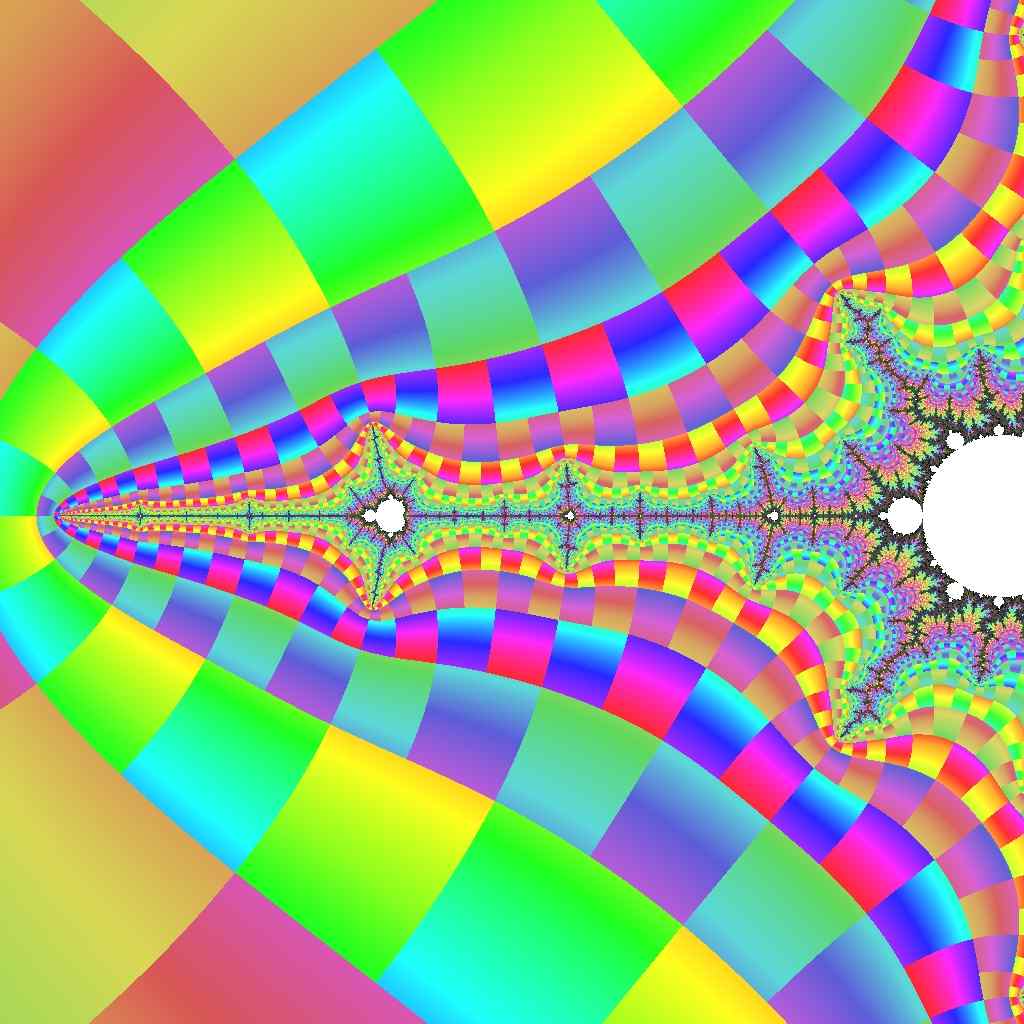
R2F(1/2B) is the R2-Name of the filament that lies on the real axis. It is the largest by overall length, including side-branches, if viewed at a low magnification and ignoring the increases in Hausdorff dimension that are evident in all filaments upon magnification. This makes it the most prominent filament for most people seeing the Mandelbrot set for the first time, and its alignment with the real axis made it appear in the very first Brooks-Matelski image. It therefore has gained many colloquial nicknames: main antenna, needle, proboscis, Spike.
It contains the largest island mu-molecule, R2F(1/2B1)S
The real axis itself, or specifically the line segment from -2.0 to the Feigenbaum Point, comprises a significant portion of R2F(1/2B). A large part of this line segment belongs to mu-molecules, but when these points are removed the result is a Cantor-like set that has positive measure (i.e. it has a non-zero total length).
R2F(1/2B) occupies the greatest range of external angles of any similarly named filament, with R2F(1/3B) and its mirror R2F(2/3B) being tied for second place; this is evident in the results of the Lavaurs algorithm. Thus it contains more islands of any given period because each such island has two external rays with denominator 2p-1 and those fractions are equally spaced over the range [0..1].
The mu-molecules on the real axis include the smallest of each period, called the R2t Series (see that article for details).
David Doff has approximated the total length of this chaotic portion of the real axis, by using the Pixel Counting method to measure pixels on a line which approaches the real axis asymptotically from above. The grid density and iterations are increased at the same time. The result is 0.55.
Acknowledgements
Measure of chaotic region: David Doff, Trinity College, Dublin
revisions: 19960208 oldest on record; 20120126 add picture; 20230525 link to R2t Series article; 20230625 clarify "largest" reasons, add Lavaurs-related paragraph
From the Mandelbrot Set Glossary and Encyclopedia, by Robert Munafo, (c) 1987-2024.
Mu-ency main page — index — recent changes — DEMZ
This page was written in the "embarrassingly readable" markup language RHTF, and was last updated on 2023 Jun 26.
 s.27
s.27