Gray-Scott Model at F 0.0460, k 0.0594
These images and movie demonstrate the behavior of the Gray-Scott reaction-diffusion system with σ=Du/Dv=2 and parameters F=0.0460, k=0.0594.
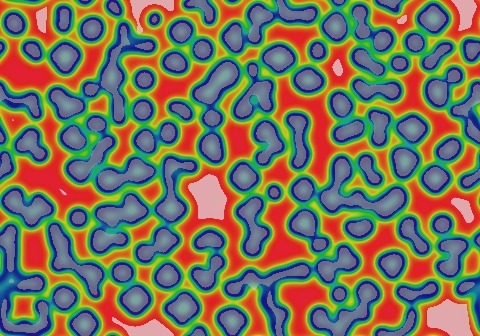
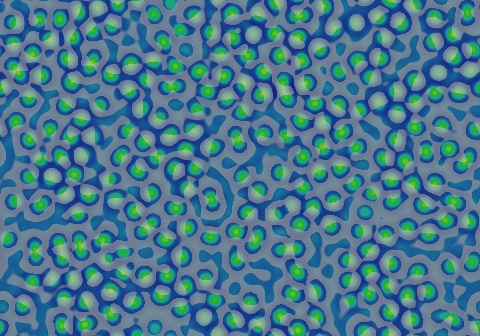
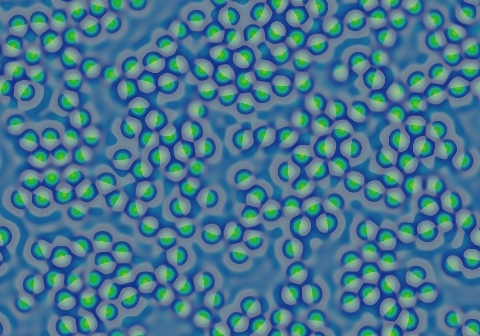
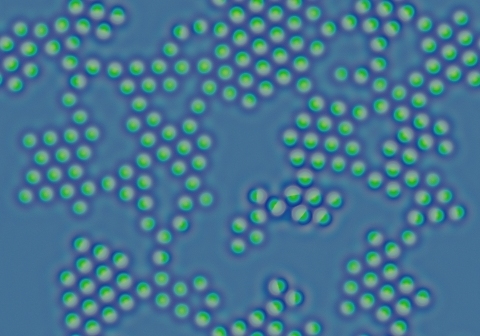
At these parameter values (which are just a bit to the right of my standard grid alignment) we see true negative solitons — areas of locally higher u (light green in these images) that retain a circular shape regardless of the width of the lower (blue) areas surrounding them.
The negative solitons (or negatons) arise spontaneously as a standing-wave pattern of concentric rings, in which overlapping waves are essential to negaton formation (evident by the fact that almost all negatons have at least two neighbors from the moment of formation). The negaton with one neighbor seen in the upper-right is viable because of multiple "neighbors" exactly two grid units away.
Ordinary noncircular negative solitons are discernable after 600 tu; almost all are circular after another 1500 tu; and drifting to form a hexagonal lattice is mostly complete by 5,000 tu. The few remaining negatons with a sole neighbor die off during the next 20,000 tu. Rearrangement completes in 50,000 to 125,000 tu.
Categories: Pearson ι; Wolfram 3 (glossary of terms)
 increase F increase F
 | |||
  |
|
15 frames/sec.; each fr. is 86 iter. steps = 43 tu; 1800 fr. total (77,400 tu) | 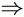 increase k 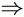
|
|

| ||
 decrease F decrease F
 |
In these images:
- Color indicates level of u, ranging from purple (lowest u values) through blue, aqua, green, yellow and pink/red (highest u values)
- Areas where u is increasing are lightened to a light pastel tone; where u is decreasing the color is vivid.
- In areas where u is changing by less than ±3×10-6 per tu, an intermediate pastel color is seen. This includes areas that are in steady state or equilibrium.
''tu'' is the dimensionless unit of time, and ''lu'' the dimensionless unit of length, implicit in the equations that define the reaction-diffusion model. The grids for these simulations use Δx=1/143 lu and Δt=1/2 tu; the system is 3.2 lu wide. The simulation meets itself at the edges (periodic boundary condition); all images tile seamlessly if used as wallpaper.
Go back to Gray-Scott pattern index
This page was written in the "embarrassingly readable" markup language RHTF, and was last updated on 2015 Nov 07.
 s.27
s.27